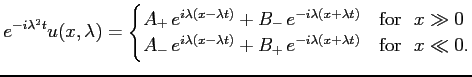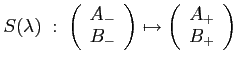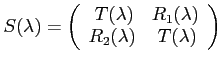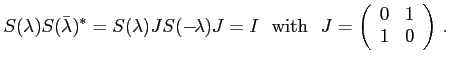Resonances as poles of the scattering matrix

We first have to define the scattering matrix. For that we will take a quantum mechanical point of view and consider the time dependent Schrödinger equation,

$$(i \partial_t - H_V) w = 0 .$$ (19)

If $u(x, \lambda)$ is a solution of (8) satisfying (9), then

$$w(x, t) = \exp(-it \lambda^2) u(x, \lambda),$$

is a solution to (19) with the asymptotics

$$e^{-i \lambda^2 t} u(x,\lambda) = \begin{cases}A_+ e^{ i\lambda... ...+ B_+ e^{-i\lambda ( x + \lambda t ) } & {\rm {for}} x \ll 0. \end{cases}$$ (20)

For $\lambda > 0$ the terms including $(x + \lambda t)$ ``move'' to the left and the ones including $(x - \lambda t)$ ``move'' to the right. Hence the terms with coefficients $A_-$ and $B_-$ are incoming (they move towards the perturbation) and the terms with $A_+$ and $B_+$ are outgoing.

The scattering matrix is defined to be the operator which maps the incoming coefficients to the outgoing coefficients, that is

$$S(\lambda) \;:\; \left(\begin{array}{l} A_- B_-\end{array} \right) \mapsto \left(\begin{array}{l} A_+ B_+\end{array} \right)$$ (21)

The matrix $S(\lambda)$ is meromorphic for $\lambda \in \mathbb{C}$ where poles with $\mathop{\rm Im}\nolimits \lambda > 0$ correspond to the square root of the eigenvalues of $H_V$. Its poles in the lower half plane coincide with resonances.

The coefficients in

$$S(\lambda) = \left(\begin{array}{ll} T(\lambda) & R_1(\lambda) R_2(\lambda) & T(\lambda) \end{array} \right)$$ (22)

are the transmission, $T(\lambda)$, and reflection, $R_j(\lambda)$, coefficients.

We have the following general properties of $S(\lambda)$

$$S(\lambda) S({\bar\lambda})^* = S(\lambda) J S(-\!\lambda) J = I ... ...m {with}} J = \left(\begin{array}{cc} 0 & 1 1 & 0\end{array} \right) .$$ (23)

Since on the real axis $\vert\det S(\lambda)\vert= 1$, the scattering phase is defined (up to an integer) as

$$\sigma(\lambda) \stackrel{\rm {def}}{=}\frac{1}{2 \pi i} \log \det S(\lambda) ,$$

and

$$\frac{d}{d\lambda} \sigma(\lambda) = \frac{1}{\pi} \mathop{\rm Im}\nolimits \frac{T'(\lambda)}{T(\lambda)} .$$

We know (see [34] and references given there) that

$$\frac{i \lambda}{T(\lambda)} = C e^{i (b - a) \lambda} \prod_{\ma... ...m Re}\nolimits \lambda_j = 0} \left( 1 - \frac{\lambda}{\lambda_j } \right) ,$$ (24)

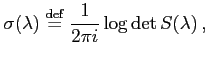
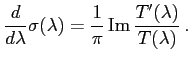
where for simplicity we assumed that there is no resonance at $\lambda = 0$. Here

$$[a, b] =$$    closure of the convex hull of $\{x \;:\; V(x) \neq 0\}$.$$$$

The convergence is guaranteed by the following consequence of Carleman's formula (see [34]):

$$\sum \frac{ \vert\mathop{\rm Im}\nolimits \lambda_j\vert }{ \vert\lambda_j\vert^2 } < \infty .$$

We then see that

$$\frac{d}{d \lambda} \log \left(\frac{ i \lambda }{ T(\lambda) } \... ...sum_{\mathop{\rm Re}\nolimits \lambda_j =0} \frac{1}{\lambda - \lambda_j} ,$$

and

$$\sigma'(\lambda) = \frac{a-b}{\pi} - \frac{1}{\pi} \sum_{\matho... ...rac{\mathop{\rm Im}\nolimits \lambda_j}{\vert\lambda - \lambda_j\vert^2 } .$$

If $\lambda_j$ is isolated from other resonances we expect from this formula that

$$\sigma'(\lambda) \simeq -\frac{1}{\pi} \frac{\mathop{\rm Im}\nol... ...ambda_j\vert^2 } , \ \lambda \simeq \mathop{\rm Re}\nolimits \lambda_j ,$$

which is a form of the Breit-Wigner formula. Note that the integral of of the right hand side in $\lambda$ is equal to $1$ and that is the phase shift at the crossing of a resonance is $1$. But one has to note that this approximation is not really rigorous without further parameters (such as the semiclassical parameter) since we do not know the size of the contribution of other terms.

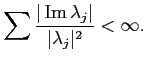
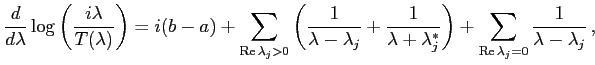
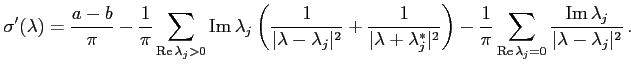
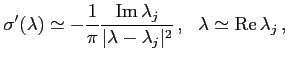
In particular, high energy behaviour of $\sigma(\lambda)$ is given by

$$\sigma(\lambda) = \frac{1}{\lambda} \int_a^b V(x) dx + {\mathcal O}\left( \frac 1 {\lambda^2} \right).$$